🫧On Bubble Rings and Ink Chandeliers

On Bubble Rings and Ink Chandeliers

published in ACM Transactions on Graphics (SIGGRAPH 2019), Jul. 2019
Marcel Padilla
TU Berlin
Albert Chern
UC San Diego
Felix Knöppel
TU Berlin
Ulrich Pinkall
TU Berlin
Peter Schröder
Caltech
Render Classic
Source Classic
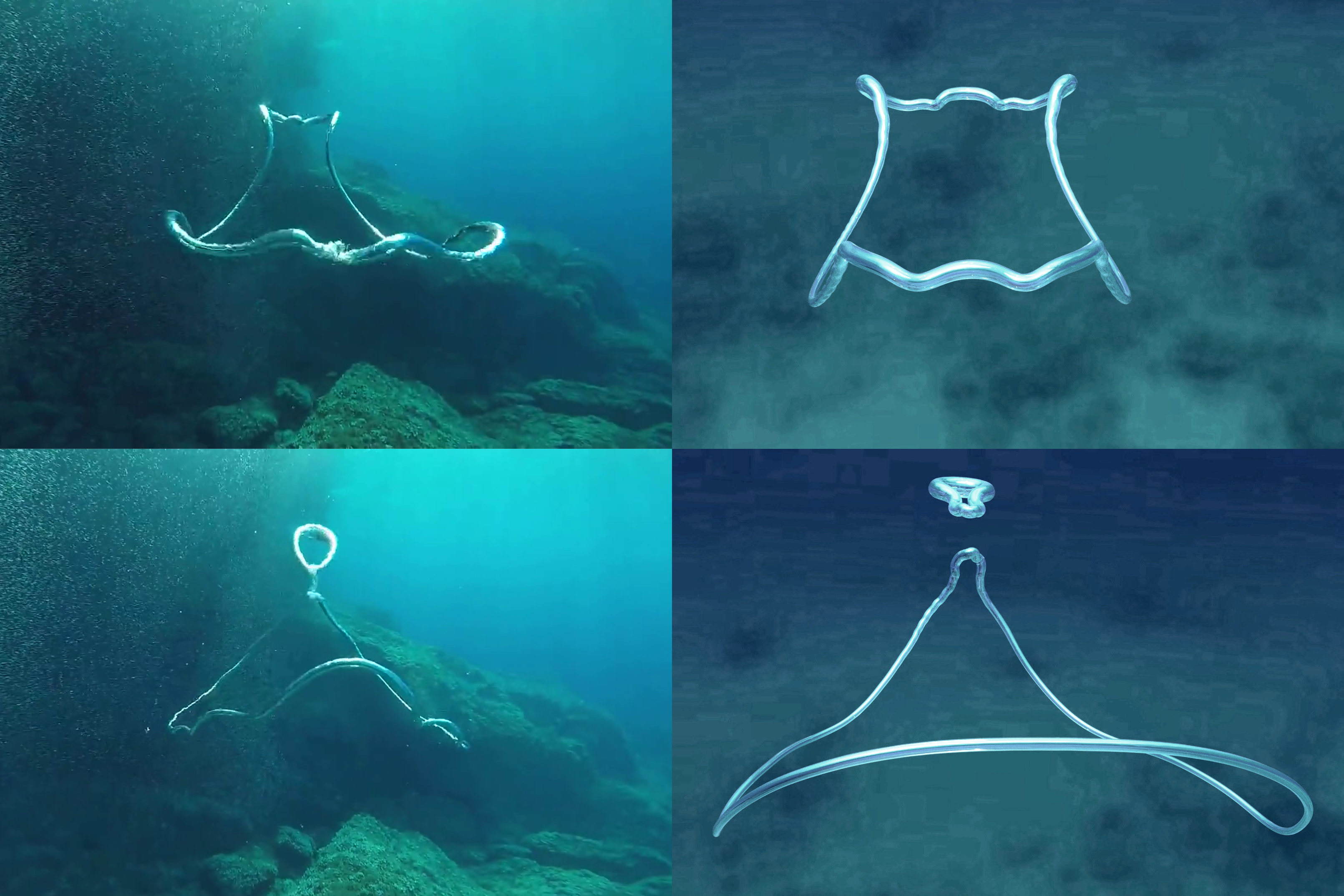
Representative Image

The reconnection of two bubble rings. Our algorithm output (left) vs real life footage.

Real-life Ink Drop
Ink Drop Comparison

Comparison of real-life ink drop (left) and the simulation output (right).

📄 See Paper 📂 Supplement Material 🗣️ Talk ✍️ Cite

Abstract

We introduce variable thickness, viscous vortex filaments. These can model such varied phenomena as underwater bubble rings or the intricate "chandeliers" formed by ink dropping into fluid. Treating the evolution of such filaments as an instance of Newtonian dynamics on a Riemannian configuration manifold, we are able to extend classical work in the dynamics of vortex filaments through the inclusion of viscous drag forces. The latter must be accounted for in low Reynolds number flows where they lead to significant variations in filament thickness and form an essential part of the observed dynamics. We develop and document both the underlying theory and associated practical numerical algorithms.

🔗 DOI: 10.1145/3306346.3322962

Submission Video

Bonus Video

Quick Summary

Talk

Acknowledgements

This work was supported in part by the DFG Collaborative Research Center TRR 109 "Discretization in Geometry and Dynamics," the Caltech Center for Information Science and Technology, and the Einstein Foundation Berlin. Additional support was provided by SideFX software.

Replicable Stamp

The supplement material of this publication has been officially verified for functionality and replicability.

BibTeX

@article{Padilla:2019:BRI:3306346.3322962,
   author = {Padilla, Marcel and Chern, Albert and Knöppel, Felix and Pinkall, Ulrich and Schröder, Peter},
   title = {On Bubble Rings and Ink Chandeliers},
   journal = {ACM Trans. Graph.},
   issue_date = {July 2019},
   volume = {38},
   number = {4},
   month = jul,
   year = {2019},
   issn = {0730-0301},
   pages = {129:1--129:14},
   articleno = {129},
   numpages = {14},
   url = {http://doi.acm.org/10.1145/3306346.3322962},
   doi = {10.1145/3306346.3322962},
   acmid = {3322962},
   publisher = {ACM},
   address = {New York, NY, USA},
   keywords = {differential geometry, fluid simulation, geodesics, physical modeling, vortex filaments, vorticity methods},
}